Numerical Treatment on a Chaos Model of Fluid Flow Using New Iterative Method

Indranil Ghosh; Md Sazzad Hossien Chowdhury; Suazlan Mt Aznam

doi:10.37934/arfmts.96.1.2535

Authors

Indranil Ghosh Department of Science in Engineering, Faculty of Engineering, International Islamic University Malaysia, Jalan Gombak, 53100, Kuala Lumpur, Malaysia
Md Sazzad Hossien Chowdhury Department of Science in Engineering, Faculty of Engineering, International Islamic University Malaysia, Jalan Gombak, 53100, Kuala Lumpur, Malaysia
Suazlan Mt Aznam Department of Science in Engineering, Faculty of Engineering, International Islamic University Malaysia, Jalan Gombak, 53100, Kuala Lumpur, Malaysia

DOI:

https://doi.org/10.37934/arfmts.96.1.2535

Keywords:

Rössler system, chaos, New Iterative Method (NIM), computational fluid dynamics (CFD), Runge-Kutta method

Abstract

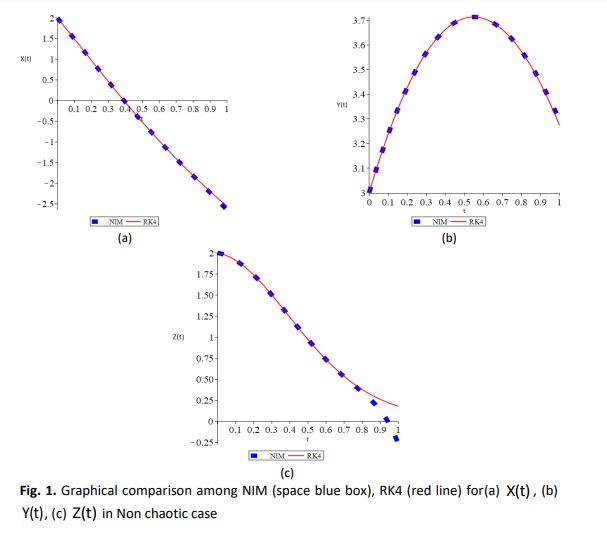
This article treats analytically and numerically to the three dimensional Rössler system. The governing equations of the problem are derived from traditional Lorentz system of fluid mechanics to nonlinear ordinary differential equations (ODEs) for modelling. A semi-analytic solution is developed by using New Iterative Method (NIM) whereas the numerical solution is presented by Runge-Kutta order four (RK4) scheme. A comparative study of the analytical and numerical solutions are made. The results confirm clearly that the two methods coincide closely for both the chaotic and non-chaotic cases of the determined system. This observation would be helpful to apply NIM on nonlinear problems of fluid flow with different fluid parameters in future.