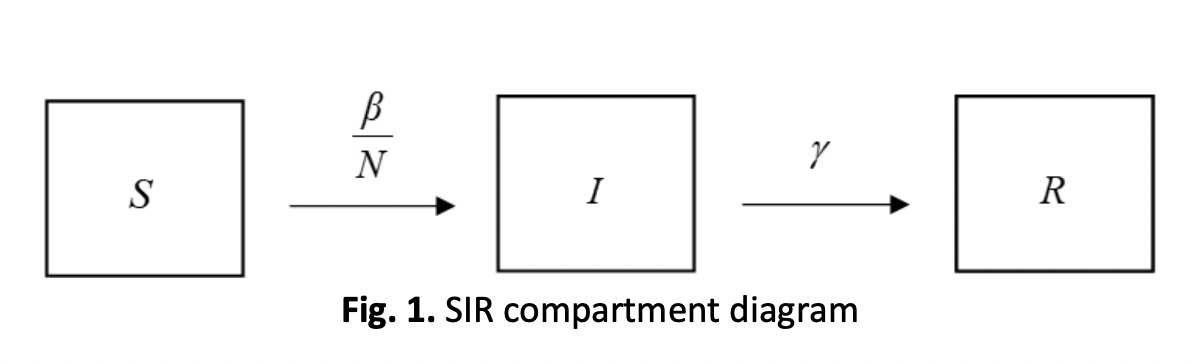
SIR Fractional Order of Simulated Covid-19 Cases using Adams Bashforth-Moulton Method

Authors

  • Zaileha Md Ali College of Computing , Informatic and Mathematics, Universiti Teknologi MARA, 40450 Shah Alam, Selangor, Malaysia
  • Fatin Nadira Rusly College of Computing , Informatic and Mathematics, Universiti Teknologi MARA, 40450 Shah Alam, Selangor, Malaysia
  • Nuramira Husna Abu Hassan College of Computing , Informatic and Mathematics, Universiti Teknologi MARA, 40450 Shah Alam, Selangor, Malaysia
  • Nur Aziean Mohd Idris College of Computing , Informatic and Mathematics, Universiti Teknologi MARA, 40450 Shah Alam, Selangor, Malaysia
  • Siti Rahimah Batcha College of Computing , Informatic and Mathematics, Universiti Teknologi MARA, 40450 Shah Alam, Selangor, Malaysia
  • Zubaidah Sadikin College of Computing , Informatic and Mathematics, Universiti Teknologi MARA, 40450 Shah Alam, Selangor, Malaysia

DOI:

https://doi.org/10.37934/araset.42.1.8292

Keywords:

Adams Bashforth Moulton method, Caputo sense, Covid-19, SIR model, Fractional calculus

Abstract

Fractional order derivative has been widely used in many different areas such as bioengineering, fluid mechanics, circuits systems, biomathematics, and biomedicine. This study introduces the system of the fractional differential equation on SIR (Susceptible-Infected-Recovered) model to simulate the COVID-19 in Malaysia. The fractional derivative is described in Caputo sense and solved by the Adams Bashforth Moulton method. The Runge-Kutta method is used to prove and validate the numerical results obtained. The graphical representations of the simulation with difference fractional order have been presented. The derivative order, with values more than 0.5 are acceptable and reliable.

Author Biographies

Zaileha Md Ali, College of Computing , Informatic and Mathematics, Universiti Teknologi MARA, 40450 Shah Alam, Selangor, Malaysia

zaile597@uitm.edu.my

Fatin Nadira Rusly, College of Computing , Informatic and Mathematics, Universiti Teknologi MARA, 40450 Shah Alam, Selangor, Malaysia

2020988367@isiswa.uitm.edu.my

Nuramira Husna Abu Hassan, College of Computing , Informatic and Mathematics, Universiti Teknologi MARA, 40450 Shah Alam, Selangor, Malaysia

2020980613@isiswa.uitm.edu.my

Nur Aziean Mohd Idris, College of Computing , Informatic and Mathematics, Universiti Teknologi MARA, 40450 Shah Alam, Selangor, Malaysia

nuraziean@uitm.edu.my

Siti Rahimah Batcha, College of Computing , Informatic and Mathematics, Universiti Teknologi MARA, 40450 Shah Alam, Selangor, Malaysia

rahimahbatcha@uitm.edu.my

Zubaidah Sadikin, College of Computing , Informatic and Mathematics, Universiti Teknologi MARA, 40450 Shah Alam, Selangor, Malaysia

zubaidah1590@uitm.edu.my

Downloads

Published

2024-03-26

How to Cite

Md Ali, Z., Rusly, F. N., Abu Hassan, N. H., Mohd Idris, N. A., Batcha, S. R., & Sadikin, Z. (2024). SIR Fractional Order of Simulated Covid-19 Cases using Adams Bashforth-Moulton Method. Journal of Advanced Research in Applied Sciences and Engineering Technology, 42(1), 82–92. https://doi.org/10.37934/araset.42.1.8292

Issue

Vol. 42 No. 1: December (2024)

Section

Articles