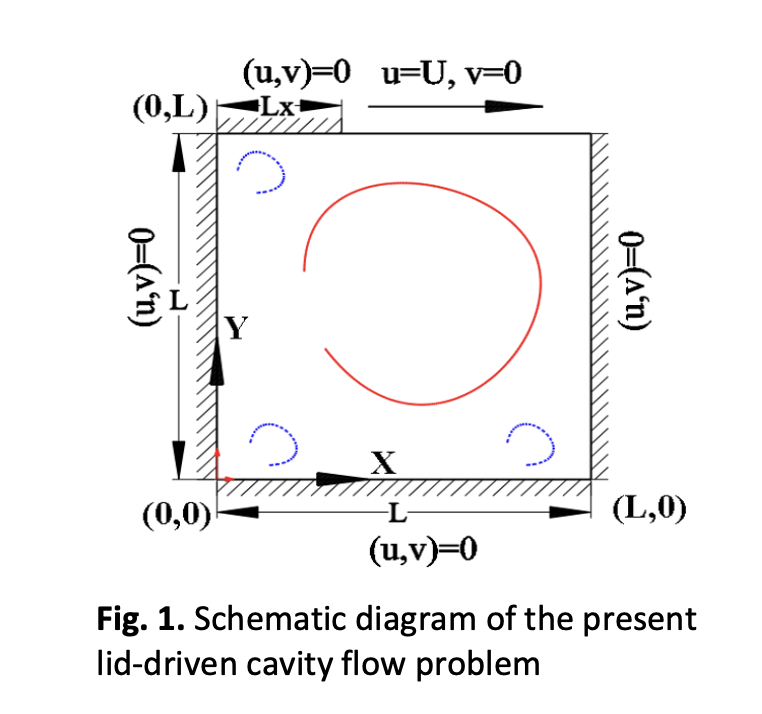
A Numerical Study on the Lid-Driven Cavity with Power-Law Fluids at Different Moving Lengths of the Top Lid

Abstract

In recent years, the lattice Boltzmann method has emerged as a promising numerical technique for solving complex fluid flow problems. This method has been successfully applied to both Newtonian and non-Newtonian fluids. Lately, the research in the field of the dynamics of non-Newtonian fluids inside a 2D square lid-driven cavity has attracted significant attention. In this numerical study, using the in-house developed Lattice Boltzmann solver, a two dimensional (2D) square lid-driven cavity with power- law fluids is investigated for different moving lengths of the top lid. Here, the top moving lid of length (L) is bisected into stationary (Lx) and moving (L-Lx) parts. The effects of the power-law index (n), Reynolds number (Re) on the flow regime, velocity and vorticity distribution in the cavity are analyzed for Lx in the range 0.0L to 0.9L. The flow regime in the cavity changes from Type-I (one primary vortex) to Type-II (two primary vortices) when the value of Lx is increased beyond a critical value. This critical value of Lx is found to depend on both n and Re. We have further investigated the inertia effects of the moving lid and analyzed the trajectory of the primary vortex center as the functions of the power-law index (n), Reynolds number (Re) and length of the moving lid.