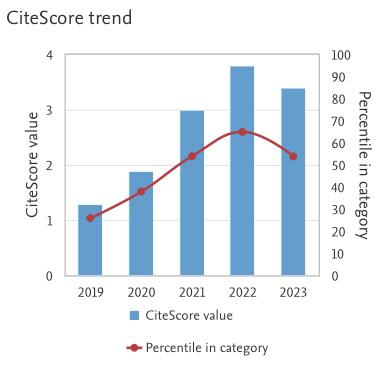
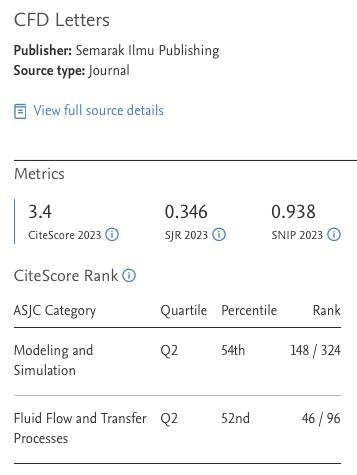
Relationship between Mesh Number and Singular Perturbation Parameter for the Solution of Singularly Perturbed Two-Point Boundary Value Problem

Abstract

There is a considerable discussion in computational fluid dynamics over the mesh structure problems for the numerical computation. Due to wide range of mesh schemes proposed by fluid dynamists, there is sometimes confusion over the correct scheme for certain problem. Furthermore, some schemes, if improperly used, can lead to nonphysical solution. We emphasize in this paper the importance of the mesh structure and singular perturbation parameter relationship in numerical solution of a singularly perturbed two-point boundary value problem. Based on the perturbation parameter, we particularly suggest a systematic technique in setting the mesh number. This is done by adopting mesh of Shishkin type. It becomes clear that the parameters of interest are linearly related. Since it is necessary to have a decision-making that is more structured, and reduce heuristic error in mesh of computational domain determination, such relationship serves as a guideline for the numerical solution of a singularly perturbed problem that is physically correct.