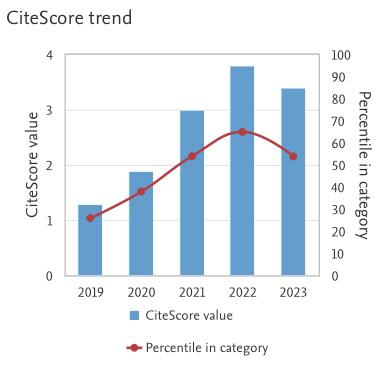
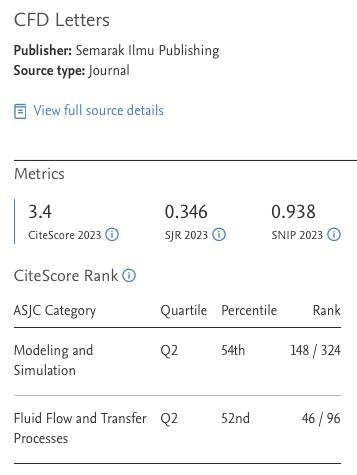
Stagnation Point Flow of Williamson Nanofluid towards a Permeable Stretching/Shrinking Sheet with a Partial Slip

Abstract

The Williamson stagnation nanofluid flow over a stretching/shrinking surface with

active and passive control are numerically studied. The main focus of the present study

is to investigate the impacts of partial slip and suction at the boundary on the velocity,

temperature, and nanoparticle volume fraction profiles and heat transfer

characteristics. It is crucial to analyze the fluid flow and heat transfer problems with

the inclusion of partial slip and suction effects due to an extensive variety of

applications in the industry. The governing partial differential equations are reduced

to a set of coupled nonlinear ordinary differential equation systems using non

dimensional variables and then it is solved using the boundary value problem solver

(bvp4c) in MATLAB. Results show that both velocity and nanoparticle volume fraction

increase as the suction parameter increases while the temperature acts in the opposite

manner. The magnitude of the reduced skin friction coefficient, the reduced Nusselt

number and the reduced Sherwood number are notably increased for the first solution

with the increasing suction parameter. It is seen that the nanofluid velocity increases

as the partial slip parameter increases whereas the temperature and nanoparticle

volume fraction of the nanofluid are decreased. As partial slip parameter enhanced,

the reduced skin friction coefficient has decreased while the magnitude of both the

local Nusselt number and the local Sherwood number are increasing. Dual solutions

exist up to a certain range of the stretching/shrinking parameter in the shrinking flow

region. The critical values of stretching/shrinking parameter increases with the

increasing in suction and partial slip effect strength suggest that both parameter

widens the range of dual solutions exist. Physically, the increment of the suction and

slip effects has delayed the boundary layer separation. The first solution is found to be

stable and physically applicable but the second solution is not based on the literature

for the similar problem presented by researchers.