Optimal Control of Transmission Dynamics of Meningitis Disease with Vaccination, Campaign, and Treatment Factors
DOI:
https://doi.org/10.37934/araset.43.2.2131Keywords:
Transmission Dynamics, Meningitis Disease, Vaccination, Campaign, Treatment, Optimal controlAbstract
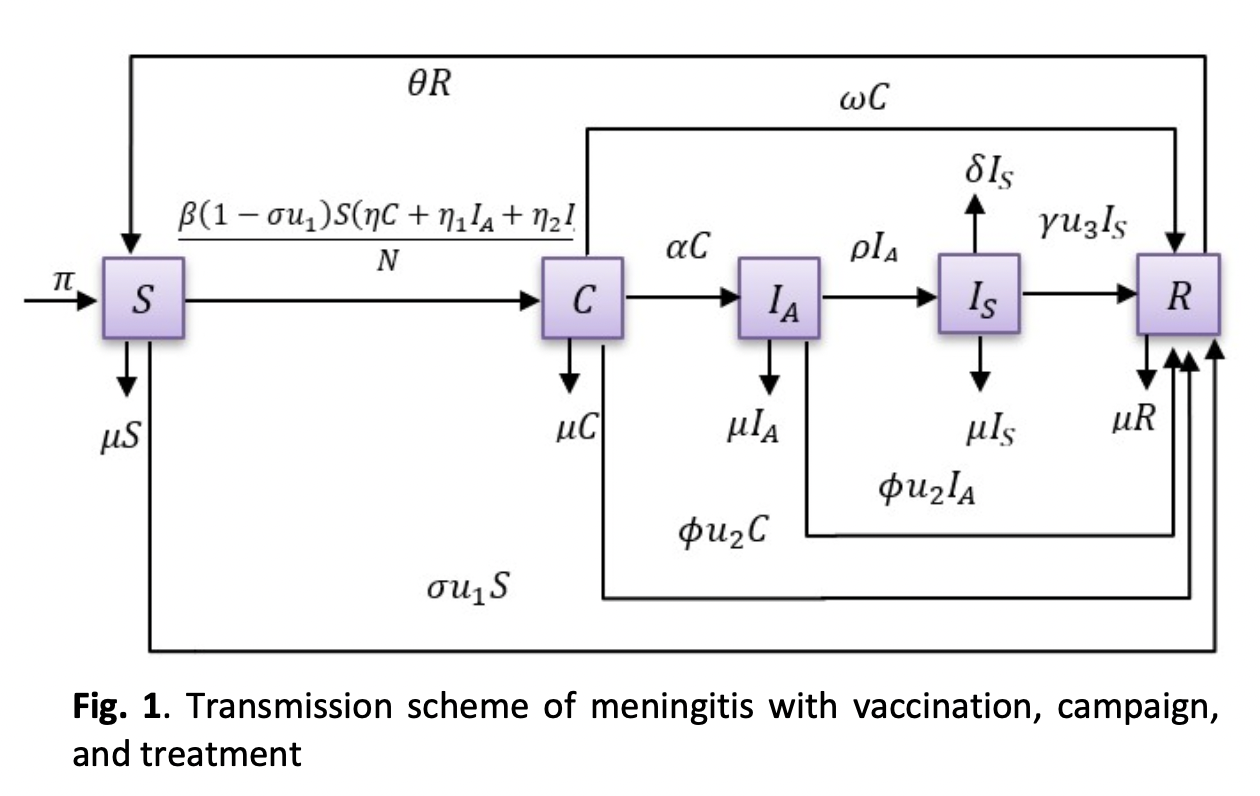
Meningitis disease is an inflammation of the membranes that protect the brain and spinal cord. This disease can be fatal to life-threatening because symptoms can appear suddenly. Meningitis is also common in infants and young children and can cause complications if not treated promptly. To prevent infection of susceptible compartment and to speed recovery of infected compartments, vaccination, campaign, and treatment are needed to prevent the spread of the disease or at least reduce the number of carrier and infected individuals. Therefore, we propose a model of meningitis disease spread consisting of five compartments, namely susceptible, carrier, infected with and without symptoms, and recovered. Vaccination, campaign and treatment are included in the model mechanism as controls. This study aims to observe the effectiveness of vaccination, campaign, and treatment in inhibiting the spread of the disease and accelerating the recovery of infected individuals. The Pontryagin minimum principle is followed to derive the optimal control problem. Numerical simulation using Runge-Kutta of order four scheme with the forward-backward sweep approach is applied to visualize the curves of state and control variables. From the numerical simulations, it is found that vaccination, campaign, and treatment are reasonable to apply in order to bridle meningitis disease from the population.
Downloads