Approximate Analytical Solutions for Non-linear Telegraph Equations with Source Term

Abstract

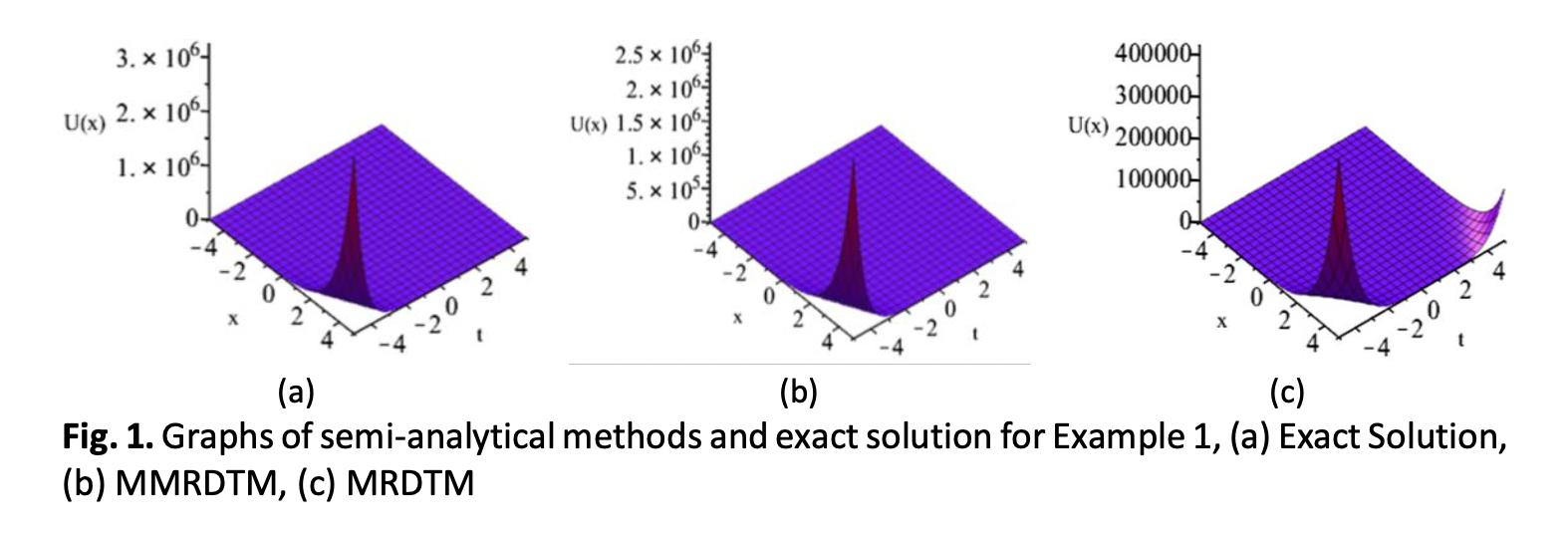
This study proposed a new version of Multistep Modified Reduced Differential Transform Method (MMRDTM) which is applicable for obtaining semi-analytical approximation on Non-linear Telegraph Equations (NLTEs) with source term. Through the experimental approach, we unveiled various advantages of the proposed method, especially its ability to analytically approximate high-speed converging series. Moreover, a significant reduction in the number of calculated terms can also be observed. Before deploying the multistep technique, the substitution of non-linear term in NLTEs with the corresponding Adomian polynomial takes place. Consequently, a simpler technique for approximating NLTEs with source term was discovered. Besides that, the solutions can be estimated more precisely over a longer time. To justify our findings, solutions of NLTEs with source term are obtained using the MMRDTM. The accuracy of the proposed method for solving these equations was tested by comparing the absolute errors between the solutions obtained by the proposed method and the Modified Reduced Differential Transform Method (MRDTM) with the exact solution. The obtained solutions evidenced that the proposed MMRDTM can deliver highly precise approximations for the NLTEs with source term.