A Mathematical Model for the Velocity of Thin Film Flow of a Third Grade Fluid Down in an Inclined Plane

Maysoon Hatem Hassan; Abdul-Sattar Jaber Al-Saif

doi:10.37934/arfmts.102.1.140152

Authors

Maysoon Hatem Hassan Maysan Education Directorate, Maysan, Iraq
Abdul-Sattar Jaber Al-Saif Department of Mathematics; College of Education for Pure Science, Basrah University, Basrah, Iraq

DOI:

https://doi.org/10.37934/arfmts.102.1.140152

Keywords:

Motion equation, homotopy perturbation method, Pade´ approximation, thin film flow, third-grade fluid

Abstract

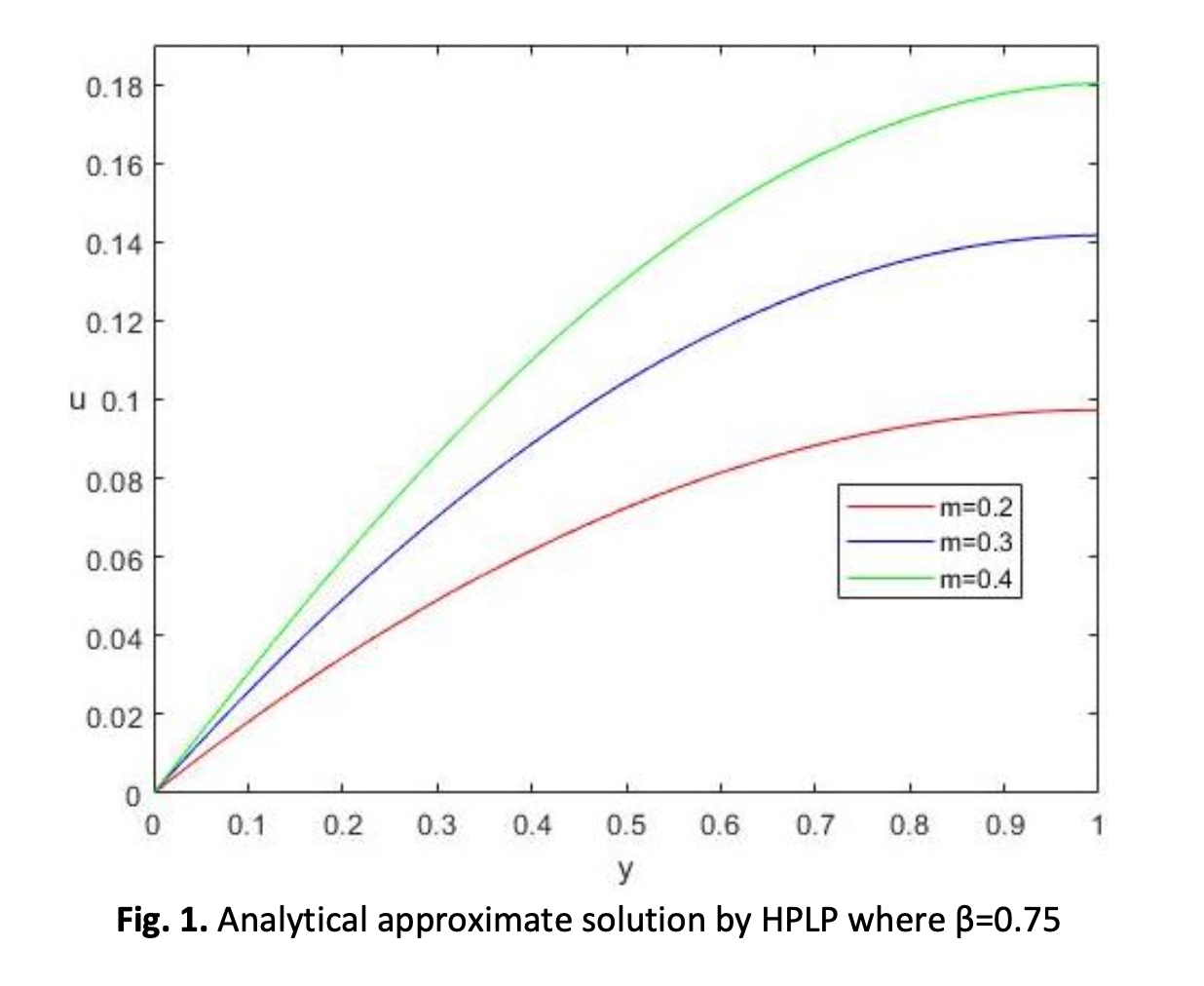
In this paper, a new technique to solve a mathematical model of thin film flow of third- grade fluid in an inclined plane is presented. This technique is based on combining the homotopy perturbation method with the Laplace transform and Pade´ approximation approach. The results we obtained showed that the proposed technique has high accuracy than other classical methods to solve this model. The measurements of error are tabulated. the validity and usefulness of the new method are derived. Moreover, the influence of parameters on the velocity profile is discussed graphically.