Application of Keller-Box Method to the Heat and Mass Transfer Analysis of Magnetohydrodynamic Flow of Micropolar Fluid between Porous Parallel Walls of Different Permeability

Abstract

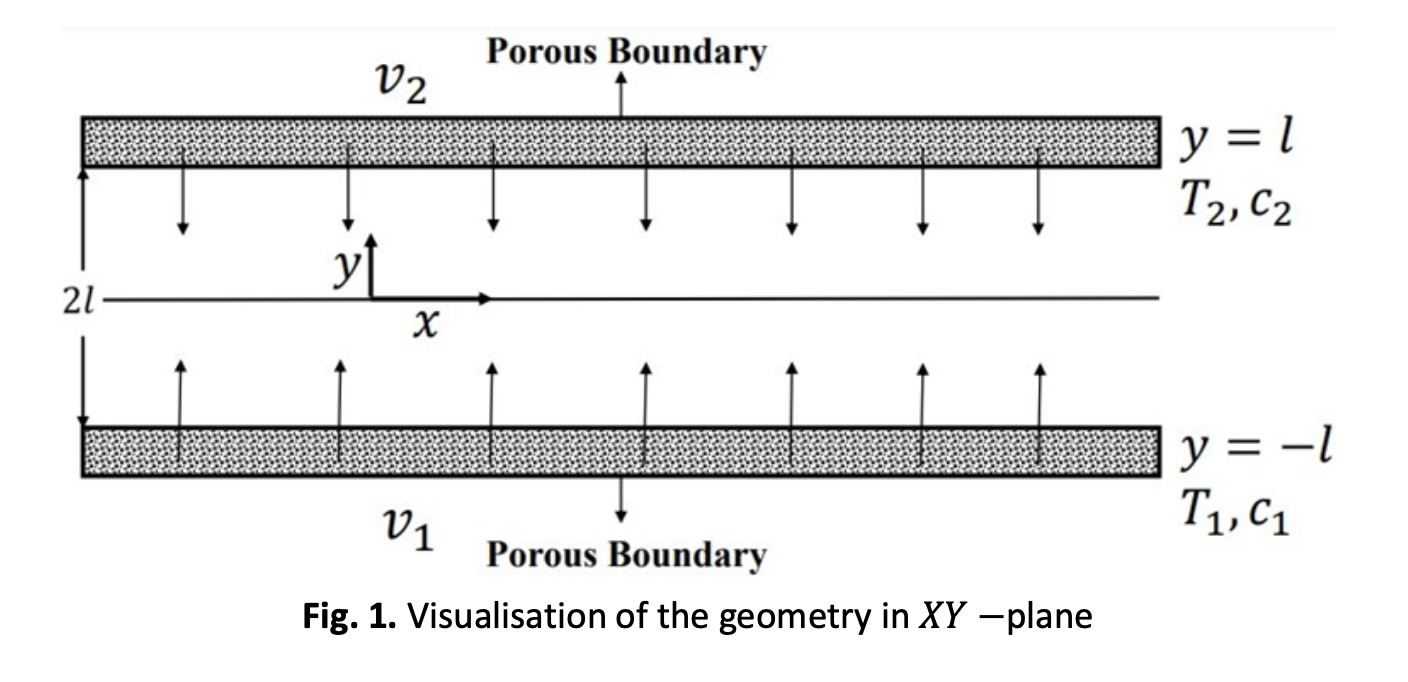
The present work seeks to investigate the problem of stable laminar micropolar fluid flow through porous walls of varying permeability. Furthermore, the effect of an external magnetic field is investigated. The resulting solution is extended to analyse the nonlinear differential equation of heat and mass transfer in the flow situation. The velocity and microrotation at the channel’s entrance are assumed to be those of Poiseuille flow. Using appropriate transformations, the governing nonlinear equations are transformed to a system of ordinary differential equations, which are then solved using a novel numerical technique based on a finite difference scheme termed the Keller-box method. The results show that the smooth and straightforward Keller-box method is an appropriate method for estimating solutions of complex governing equations since it is simple to implement. Graphs and tables are used to explain the effect of significant parameters such as the suction Reynolds number, micro rotation/angular velocity parameters, and Peclet number on the stream function, temperature distribution, and concentration properties of the fluid. The effect of wall permeability on longitudinal velocity and microrotation has been investigated. The couple stress and skin friction on the walls have also been calculated. The effect of variation in Reynolds number is showing the importance of inertial forces in comparison with viscous forces. The velocity boundary layer is observed to decrease when there is an increase in Reynolds number. Furthermore, the accuracy and convergence of the obtained solutions show that the Keller-box method is applicable to various nonlinear physical problems, even those with significant non-linearity.